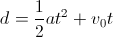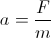Assuming identical and sufficient ABS, and ignoring aerodynamics, are tires the only factor for braking distance?

Question

I had my first accident two days ago (minimal injuries so don't worry about that), and it's got me thinking about a lot of different aspects to driving and cars, such as braking. Had my braking been even slightly less effective, it would have been a very different story.

This lead me to do research on cars with good 60 mph - 0 mph distances, but in doing so someone claimed that it was effectively just a tire comparison.

So is this true? Assuming brake pads which are able to lock up the tires and ABS, and ignoring downforce, is it all just down to the tires?

To specify the scenario, let's say it's a one-off (i.e. normal temps) hard brake from 60 mph to 0 on asphalt. Let's consider both a perfectly smooth road, and a slightly bumpy road. Ideas on other potential factors:

• Weight of car - physics tells me that friction is proportional to the normal force, so I figure this doesn't matter
• Suspension stiffness - this would lessen the front dipping, but does that even affect braking distance?
• Suspension rebound - a poor rebound would possibly cause momentary airtime if the road is bumpy, would this be substantial?
• Chassis rigidity - would a chassis that warps a bit more under heavy forces cause some tire skipping or something?
• ABS quality - ignoring EBD, is there a substantial difference in the effectiveness of ABS between car models? My '94 3-series BMW doesn't seem to shudder like my parents' '02 Corolla. At a guess, shuddering may be brief moments of the tires skidding or of the brakes releasing - neither would be great.

I know EBD (Electronic Brakeforce Distribution) might be a help, but I may just put that in another question.

Answer 1

tl;dr: No.

This sort of vehicle dynamics question best addressed by Racing Car Vehicle Dynamics

What follows is a basic discussion at the high school physics level. As you will see from the reference text, high school physics is insufficient to statically model the complete vehicle system. A dynamic model is required to agree with easily obtainable experimental data.

Assuming brake pads which are able to lock up the tires and ABS, and ignoring downforce, is it all just down to the tires?

No.

Weight of car - physics tells me that friction is proportional to the normal force, so I figure this doesn't matter

Incorrect. Mass is the dominating factor.

Your critical equation here is:

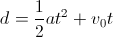
where

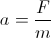
d is your stopping distance and, as you can see, it is defined in terms of your acceleration a (deceleration in your case), your initial velocity v_0 and the time required to get to speed zero t. F is the decelerating force of the entire vehicle and its braking system while m is the mass of the vehicle.

For completeness: to solve for t, you're best off using the energy equations. However, the above two equations are sufficient to do a numerical approximation in the spreadsheet of your choice.

So, all other things being equal, you can easily see that the mass of the vehicle dominates the entire system. If you change nothing else, a lighter vehicle stops faster (higher acceleration for the same force). Two identical cars with a different number of passengers will slow at different rates.

Experimental confirmation: All of this discussion is fun but solid physics should be confirmable (or falsifiable) using an experiment.

Materials required:

1. A fully fueled car.
2. A measured course for 60 mph braking tests (i.e., a straight track, a Brake Here sign and 10 meter markers).
3. An additional load of 20 fifty pound bags of sand.

Procedure:

1. Perform ten braking tests from 60 mph without the additional load. Measure the distance required to stop on each run. Note that back-to-back runs will likely induce brake fade (the distance will increase with brake temperatures).

2. Refuel the car.

3. Add 10 fifty pound bags of sand to the vehicle (for a total of 500 additional pounds). Repeat the same braking tests from 60 mph on the same course. Note the stopping distances. Note that the distances required to stop are longer. Note that back-to-back runs are resulting in increasingly severe brake fade (i.e., those long stopping distances are getting longer much faster).

4. Refuel the car.

5. Add 10 more fifty pound bags of sand (for a total of 1000 additional pounds). Repeat the same braking tests. Note that stopping distances required are much longer. Note that back to back runs are resulting in brake fade much more quickly (possibly reaching a state of terrifying as the brakes seem to cease to function).

Conclusion:

Returning to our original equations, we can see that as m increases, there is a distinct increase in the distance d and time t required to stop. If F were a pure kinetic or static frictional force, we would expect it to increase linearly with m, resulting in nearly identical stopping distances.

As this is not the case at all, we can conclude that F is not an idealized frictional force.

So assuming the same tires, and brakes that can hold the tires at that maximum static force, I don't see why mass would increase braking distance.

Yes, the situation would be very simple if a car were an ideal solid block from a physics textbook (i.e. a spherical chicken). In your comment, you're substituting m in several places as if it were always the same on every wheel. This would be the case if we were talking about a physics problem. Unfortunately, an actual car is a hollow box sitting on springs, riding on round balloons made of rubber and steel.

When you mention the normal force, you're talking about the force of the tire patch on the road. This situation would only match the basic friction function if the vehicle were non-moving with locked brakes. There's an amount of force that's going to be required for me to push this heavy thing across the parking lot (with tires screeching the whole way, I assume). Heavy car => harder to push.

Unfortunately, none of the above is actually relevant.

The reality is that the normal force used for kinetic friction to stop the car isn't the tires. It's actually the force of the brake pads on the rotors (or drums but I'm assuming disk brakes for the sake of having something easy to point at). Their clamping force is what actually slows the wheel. That only results in slowing the vehicle if the tires are statically coupled to the surface. A wheel that stops spinning is skidding, not braking.

Suspension stiffness - this would lessen the front dipping, but does that even affect braking distance?

Suspension rebound - a poor rebound would possibly cause momentary airtime if the road is bumpy, would this be substantial?

Chassis rigidity - would a chassis that warps a bit more under heavy forces cause some tire skipping or something?

Anything that increases the tire contact patch will increase the ability of the road to induce a torque on the wheel (and vice versa). A big patch will grip the road well, keeping the wheel spinning and allowing the brakes to use more gripping force before the wheel stops, skidding the tires. A smaller patch grips poorly, resulting in a skid with much less gripping force.

This is where your ABS system attempts to optimize a bad situation: it tries to keep all the wheels from stopping by relaxing and re-gripping the clamping force of the brakes near the limit of traction. This modulation of the brakes is exactly what a skilled driver will attempt to duplicate.

Why is it worse to have the majority of the load on two tires instead of four?

Again, the braking system is the totally reliant on the use of the brake disks and the clamping force that it can apply before the wheels lock. As the weight leaves the back tires, their contact patch approaches zero. As a result, the braking system cannot apply much clamping force before those wheels lock (i.e., they're now out of the picture).

However, shifting the weight forward has increased the contact patches of the front tires but they haven't doubled (this is a consequence of many things, including that each is an air-filled balloon that doesn't spread linearly with an increase in the weight it supports). As a result, each front tire is now receiving less than two times the torque that each tire was receiving before the weight transfer. Consequence: each front rotor can be gripped with a higher force but not double the force, resulting in reduced braking and longer stopping distances.

ABS quality - ignoring EBD, is there a substantial difference in the effectiveness of ABS between car models?

This can really only be answered in terms of a specific situation. What is the scenario? What tires are in use? Snow vs. ice vs. sand vs. rain? Cold or hot tires?

In general, any modern ABS system is better than none. It is close to optimal when compared to the average driver, their situational awareness and their reaction times.

Can a very skilled driver brake better without ABS than they would with ABS?

I firmly believe that Michael Schumacher could out-brake me on the track, in the same vehicle, no matter what ABS system I were to use.

So what?

Unless you're a champion Formula One driver (or an effective equivalent), I put it to you that this is a meaningless comparison when you talk about driving in the real world, on real roads with real people who aren't paying nearly as much attention as they should.

Answer 2

Preface

I ended up doing a fair bit of thinking and research on this, so I figure I may as well write up what I found. Thanks all those who responded, particularly BobCross. In the end though, I wanted an answer that went beyond calling a car a mystery box on balloons - I asked this question because I actually want to understand it.

Intro - Tires

Given the situation of a car that has sufficient braking power to lock the wheels and ignoring aero, tires are the final determinate of stopping power. Having said that, there's a lot of factors that affect how well those tires grip the ground.

Weight of a Car

The stopping power of a car is friction. With proper ABS, the wheels are rolling in contact with the road, so we're primarily talking about static friction. Some slip occurs because of the softness of tires, but it's pretty close to static.

Basic physics tells us that friction is equal to the normal force times the coefficient of friction, i.e:

Ff = µ * Fn

where Ff is the force of friction, µ is the coefficient of friction, and Fn is the normal force.

However tires are a fair bit more complicated. For one, the coefficient of friction decreases as the normal force increases (see this Wikipedia article for more details). Below is data taken from that article:

Vertical load   µ
(lbf)           max
900             1.10
1350            1.08
1800            0.97

The equation from before tells us that stopping distance is inversely proportional to the decelerating force, so with the above data, a doubling of mass results in a -12% decrease in µ, and therefore a +14% increase in stopping distance. Why? This equation:

v_1^2 = v_0^2 + 2ad

i.e. given a start and finish speed, acceleration is inversely proportional to distance.

So more weight results in a longer stopping distance, but the relationship is only slightly positive, and definitely not linear.

Note: Increasing weight puts the car at risk of worse brake fade, but that's out of scope of the question

Suspension

In regards to braking, suspension is important for:

1. Keeping the tires planted on the road
2. Keeping the distribution of weight even across the tires. Uneven distributions of weight, such as in the case of nosediving, decreases overall braking ability as above we see that grip doesn't increase linearly with load.

Stiffer suspension definitely helps with (2), however (1) simply requires an ideal stiffness/rebound setup for the road - whatever keeps the tires best planted. For quality asphalt, a stiff and fast setup will probably be best.

Chassis

All the chassis needs to do for braking is act as a rigid body between the suspension, so the suspension can do its work. So it can make a difference, but it's not going to be major unless the chassis really sucks.

ABS

EBD is part of the ABS system, so you can't really analyse good vs bad ABS without considering the gains from EBD.

A good ABS setup can apply different levels of braking to different wheels, so it's possible for ABS to out-brake a human, simply through having more controls. Though on quality asphalt, varying left-right braking balance is probably not as significant.

It's hard to say whether there really are poor ABS systems out there, but it's entirely possible.

Conclusion

Tires definitely are the money maker when it comes to braking, if you already have a powerful brakes with good ABS, however there are other factors that play a very significant role.

And if you step outside the scope of a one-off brake ignoring aero, well then... you have aero to consider as that's huge for high speeds, and that assumption of brakes locking up the tires is no longer a given after many aggressive track laps with a car pushing out several hundred kW. Where's all that power going to go?

Answer 3

One point to remember and bear in mind is that the main criteria in all of this is the coefficient of friction of the tyre. This affected by the tyres pressure. If you were to have a given vehicle on a cold flat highway with good tyres at correct pressures your braking would be optimal. Any variation from this, argumentatively ideal situation, would detract from the braking performance. Road conditions, tyre quality, pressures, weather and vehicle state of repair all have their influence. ABS systems are usually manufactured for the car manufacturer, this means you have the same system on very many makes and models. All the ABS systems you will find will be indistinguishable from each other. Price of the systems will be a difference, as some maufacturers will import components that they have exactly made for them in countries where labour and health and safety concerns are less so making them cheaper. (VW do not make their own gearboxes, they are made in Japan.) Micheal Schumacher had the benefit of telemetry, GPS, and Ross Brawn, as well as un-resricted access to the racing arena to practise to perfect his braking. Oh yes, he did have a little talent as well :-).

Answer 4

The short answer is that the following things all play a role in stopping distance:

1. The weight of the car,
2. The "grip" of the tire. Softer and wider (and a little under-inflated) is better.
3. The proper distribution of braking across all tires. In an ideal scenario, all four tires will be given an equal amount of braking work (but see the next point)
4. the degree to which each wheel's burden is similar to the others. When you brake, the car's center of mass shifts forward. This means that your front brakes do between 60% and 80% of the braking. If this can be made more 50/50, it would help.*
5. A suspension+shock set up that will keep each wheel in contact with the road as much as possible.

*WRT #4: it is extremely impractical to set up your car's suspension components to keep the center of mass in a 50/50 distribution during braking. I don't think it's even physically possible. But if it was you would have an extremely hard and uncomfortable front suspension, whilst the back would be marshmallows.