Why does stopping and starting a car in heavy traffic burn more fuel?

Question

Why does stopping and starting a car in heavy traffic burn more fuel than simply coasting on a highway at 55 mph?

Answer 1

If you think about what the car is doing in both cases you'll see why you burn more fuel when accelerating.

General theory

F = mA (Force is equal to mass times acceleration), and in this case the force is applied by the engine. The more force, the more fuel is burned.

Acceleration

In stop and go traffic, you are making frequent stops, and accelerating from zero to some relativly low speed, like 30 MPH. Per the above equation, (F=mA) you must have a force in the direction you want to accelerate the mass of your car. But that's a net force. You have the force of the engine moving you forward, but you are being resisted by inertia, friction, and at some point even the air resists your attempt to accelerate. The engine must overcome all of these forces by applying a bigger one. More force is more gas burned.

Highway coasting

While coasting on the highway you are maintaining an acceleration of zero. So the net force applied is zero. So, you only have to match, not exceed like when accelerating, the forces of friction and aerodynamic drag. Less force, means less gas burned.

I hope that helps!

Answer 2

Every time you brake, the energy is wasted. Brakes convert mechanical energy of a moving car into heat via friction (they heat up). This is where the energy is ultimately "lost". Then, when the traffic moves forward a bit, you of course need to accelerate - and this is where you actually use gas from your tank to put this energy into getting your car to move.

When you coast at constant speed, the only big energy losses come from air resistance. This resistance depends on speed and shape of your car, so with moderate speed (like 55mph) and modern, aerodynamic car you actually lose less energy than from repeated braking in a traffic jam. Of course, if your car is less aerodynamic (eg. carries big baggage on the roof) or you drive it very fast, you will eventually reach a point when you'll burn more fuel coasting than in traffic jam.

(I skipped energy losses in rubber tires, because they remain mostly the same. Also, if you can coast in 10 min but spend full hour in a jam, that's lots of idling - but idling is not as important as all that braking.)

This also explains why vehicles with electric motors are much more efficient at such start-stop traffic - instead of regular (friction) braking they do "regenerative braking" instead and get some of the energy back into the battery.

Answer 3

Your engine is always burning gas when the car is running.

When you're stationary, you are burning gas to keep your engine running, without actually moving the car, so you're actual miles per gallon (MPG) at that moment is 0.

When you begin to accelerate, you are using more gas than when the car was idling, but then you have to press the brakes, essentially wasting the extra gas you just used to get up to speed.

Once you're up to speed and no longer accelerating on the highway, the engine is only using 20-40 horsepower to maintain that speed. When you're cruising at 60 mph you're covering mile a minute, so depending on the car, your relative fuel consumption is much higher.

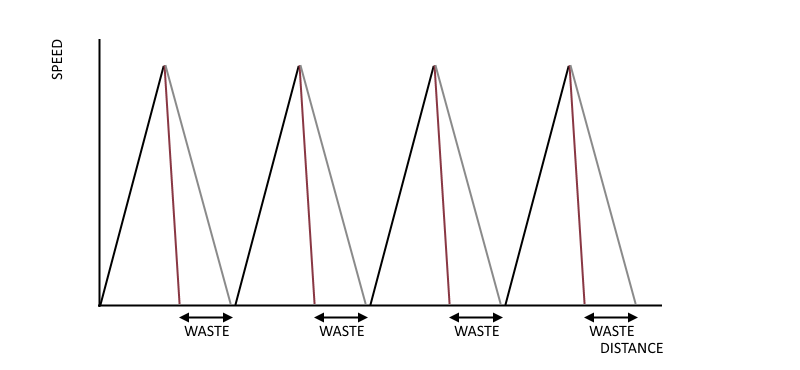
The graph below displays the Brake Specific Fuel Consumption (BSFC - brake specific meaning the engine was mounted on a certain style of engine dyno, rather than in a car). The fuel consumption is measured in grams per kilowatt-hour (1 KWH = 1.34 horsepower). The maximum torque vs RPM (engine Revolutions Per Minute) is displayed at the top of the graph (black line w/ black dots). As you can see, least amount of fuel per KWH is used when THIS engine is running at 2-3k RPM, and outputting 80% of max torque.

Again, when cruising, you only need a fraction of your total horsepower. The engine rpm for most cars in top gear at highway speeds is usually 2500-3500 RPM, so even as your torque requirement goes down and you fall out of the optimum fuel efficiency range, when the value of the denominator (power needed to cruise at 60) decreases, as does the numerator (amount of fuel used).

Answer 4

The most important aspect of the answer to this question is found in Newton's first law of motion:

An object at rest stays at rest and an object in motion stays in motion with the same speed and in the same direction unless acted upon by an unbalanced force.

This is the same reason that space shuttle use something like 90% of their fuel on take-off.

As cdunn went into, it's all about force (F). More fuel/s = more force/s.

The key to understanding it is that little snippet "unless acted upon by an unbalanced force."

In the case of your example of a highway with ups and downs, gravity comes greatly into play. On the decline g becomes a positive force. To illustrate clearly I'll use extremes.

Say your decline is 90 degrees, or vertical. That means that g (10m/s^2) is added to the power of your engine. This is why vehicles have intentional methods of engine breaking and drag in various parts - so you don't just blast down hills. Conversely, when traveling back up this Gravity is now a negative force on your engine. So you either need to produce more force from the engine, or produce more force via inertia.

Extreme Example

Say the following is true:

    motor output (Mo)= 250 HP or ~ 19,020 kg-m/s^2

    curb weight (cw)= ~1800 kg

    g = 10m/s^2 • cw = ~18,000 kg-m/s^2

    friction = 0

    surface resistance = 0

Using -- t=(v-v0)/a -- we get the following.

In this case nothing is in play except
gravity and motor output. Which
means that in a dead fall you have 
~37,020 m/s^2 for and in a vertical 
incline only ~1,020 m/s^2. 

So on the decline it only takes 
0.00075 seconds for the car to reach
100 km/h. 

Whereas on the incline, it takes 
0.0272 seconds to reach the same 
speed.

While this may not look like much, you can see it's a huge difference.

True that attempting to maintain a constant speed where there are hills is not the most efficient (I cut how most cruise controls systems handle hills). But on flats it is. The trick with hills is to equalize your forces. Getting to a proper speed on a down hill will allow your inertia to carry your farther up hill without massive input from your motor.

But hills aside - you initial question is "why does stopping and starting in traffic burn more fuel." The answer to that is simply because of inertia. But! There are additional actors as well. For example, sitting stopped. Your motor is burning fuel and you are not traveling. So your not really getting 0 MPG, but more like -x MPG because it brings the overall MPG of your trip or count down to eventual 0 or even a negative ratio (e.g. 15 Gal./1 Mile).

Variables like wind resistance, drag, inefficiencies and gravity don't even really come into play until there flowing traffic.

Answer 5

Any engine can not have 100% efficiency; there are always energy losses.

When cruising on highway you generally use the top gear and many cars are tuned to have peak efficiency there. In that case your energy losses are due to aerodynamic drag, tire rolling and engine and transmission friction. Note that first two ways are proportional to squared veocity, transmission losses are proportional to velocity and engine friction is proportional to the actual RPMs.

When stuck in traffic jam you usually go on first two gears only leading to lower drag but higher engine friction and the engine operates in wide range of RPMs. When you brake to stop, all the kinetic energy, you got from the fuel, is wasted; when you stay with engine on you waste the fuel just to keep the engine on. If you accelerate you burn more fuel to raise the kinetic energy, if you shift too early or too late you burn extra fuel just because the engine is out of its optimal RPM range. When starting from halt you have to slip the clutch for a while; another waste of energy.

Even though you do not brake to stop at all (wasting your kinetic energy), you do use engine braking, you do use start-stop, you shift at right times; you cannot reach the fuel economy when cruising smart way.

Answer 6

Another way to view this is to visualise throttle opening.

When you're cruising, the pedal is held down to some position more than idle, but less than maximum

When you're taking off and accelerating, the pedal is pressed down further, which opens the butterfly valve allowing more fuel/air mixture into the engine.

Hence more fuel is used to accelerate than to cruise.

Yes I realise answer is fudging, modern cars, computers, injection etc - handwave and simply

Separately, idling uses fuel for no progress, which is why some cars shut off their engine at a dead stop. As a cyclist it sounds so strange at the green light, to hear three or four cars all turn over their engines at once.

Answer 7

Simple answer: fuel burn at cruising (at a steady 55 mph) is proportional to the friction (aerodynamic \ tire \ mechanical bearings). High transient driving (stop-and-go with conventional friction braking) energy consumption is significantly higher than energy burn due to steady-state friction. Hybrid electric braking is energy conservative and should be thought of as a special case.

Wear and tear on the engine / tires / brakes are also pronounced in cars that are driven in stop-and-go roads.

Answer 8

To cut it very simple: acceleration costs energy. Braking does not win you any energy (in your average car at least).

Hence, if scenario 1 involves accelerating and braking, and scenario 2 involves a steady cruise at constant speed, then scenario 1 will cost more energy (fuel), simply because you spend the fuel for acceleration. It's not the braking that is inherently bad, but having to brake is telling you that you could have avoided the acceleration in the first place and thus saved the acceleration fuel usage.

Addendum: there is a scenario 3: accelerate to your target speed as quickly as possible in the appropriate gears, then disengage the clutch and roll with the motor at idle. This uses even less fuel than scenario 2 because the average motor will be more efficient at higher RPMs (up to a point, don't press the gas pedal all the way to the floor since modern motors will then pump additional fuel in to give you kind of an "afterburner" effect).

This needs some practice to get right, i.e. you have to accelerate up to a high enough speed that you get a meaningful amount of rolling time, while not breaking speed limits and not hindering other cars; also it does not really benefit you if you then still have to brake at the end of the roll. So, I'd not advise newbies to do that, but experienced drivers can get a few percent of fuel savings out of it. Google "hypermiling".

Also, in general, try to brake with the motor instead of the brakes (if safety allows it), obviously, hence the motor will use 0 fuel (instead of the miniscule idle fuel) when you do that.

Answer 9

One reason is that fossil fuel engines are tuned to run most efficiently around 50-60mph, so any other speed will not deliver as much torque for the fuel being burned - that's why cruising speed is where it is.

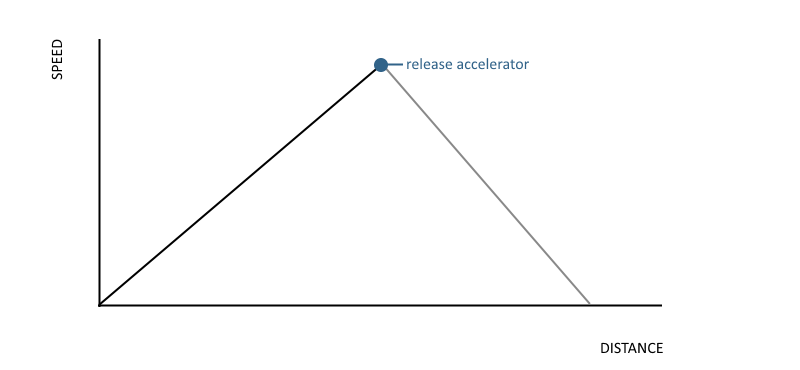
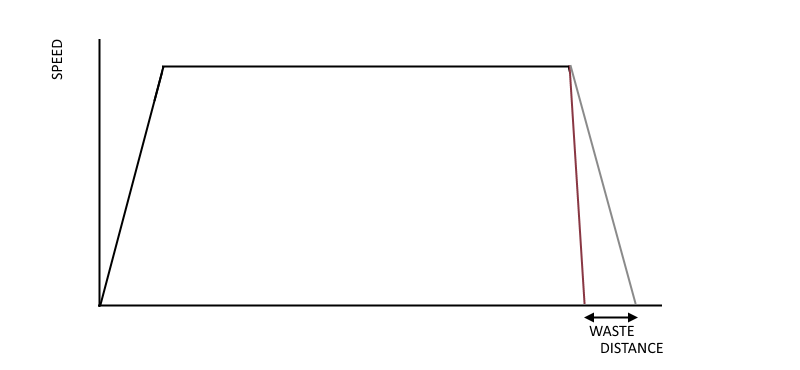
Another, which I will focus on, is that regardless of what speed you travel at, every time you brake, you waste energy. Here's what it looks like if you accelerate and then take your foot off the accelerator:

Here's what it looks like if you hit the brakes:

And a comparison:

Thus any time you brake, you haven't gone as far as you could've - you've spent fuel up front in accelerating that could've taken you further. You now have to spend energy again to cover that distance.

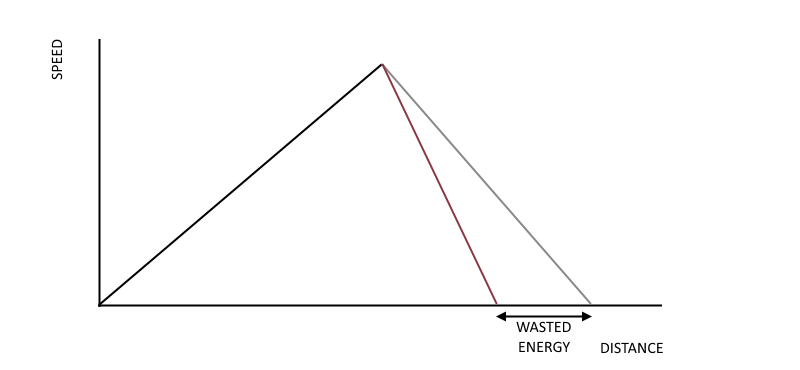
Here's what that looks like in traffic - notice the accumulation of wasted energy:

Verses the waste if you just brake once at the end:

Incidentally, this is one problem hybrid cars address: when you hit the brakes, they use induction to recharge the battery, and there's less waste.

Answer 10

I think we can simply refer to Newton's first law of motion in Physics to answer this question in the most simplest way.

Newton's First Law of Motion: I. Every object in a state of uniform motion tends to remain in that state of motion unless an external force is applied to it. This we recognize as essentially Galileo's concept of inertia, and this is often termed simply the "Law of Inertia".

When we consider how this applies to a car, a car coasting along a flat surface will continue at the same speed unless some force acts upon it. (Ignoring drag and friction of rolling along the road for this example).

With a stationary vehicle, you need to burn fuel to create the force that acts upon the car and its components (engine components, driveshaft, road wheels and the like) to speed their rotation up and accelerate the vehicle.

Using the brakes applies a strong frictional force on the car, converting the inertia (kinetic energy) of the car into heat.

In a car that is stopping and starting, you are burning more fuel because you lose kinetic energy stopping as waste heat, and then have to spend energy from fuel to increase the inertia of the vehicle and its components again when you accelerate.

Therefore a car that is stopping and starting uses more fuel.

Answer 11

I would argue driving in stop and go traffic uses less fuel than driving at freeway speeds.

Consider the following scenario using typical highway and stop-and-go speeds, and realistic MPG at those speeds. You can see the car burns fuel at a quicker rate on the highway than in stop-and-go traffic.